Rates of Decay, Radiodating
12.1 Radioactive Decay
Learning Objectives
- Calculate kinetic parameters for decay processes, including half-life
- Describe common radiometric dating techniques
12.1.1 Radioactive Half-Lives
Radioactive decay follows first-order kinetics. Since first-order reactions have already been covered in detail in the kinetics chapter, we will now apply those concepts to nuclear decay reactions. Each radioactive nuclide has a characteristic, constant half-life (t1/2), the time required for half of the atoms in a sample to decay. An isotope’s half-life allows us to determine how long a sample of a useful isotope will be available, and how long a sample of an undesirable or dangerous isotope must be stored before it decays to a low-enough radiation level that is no longer a problem.
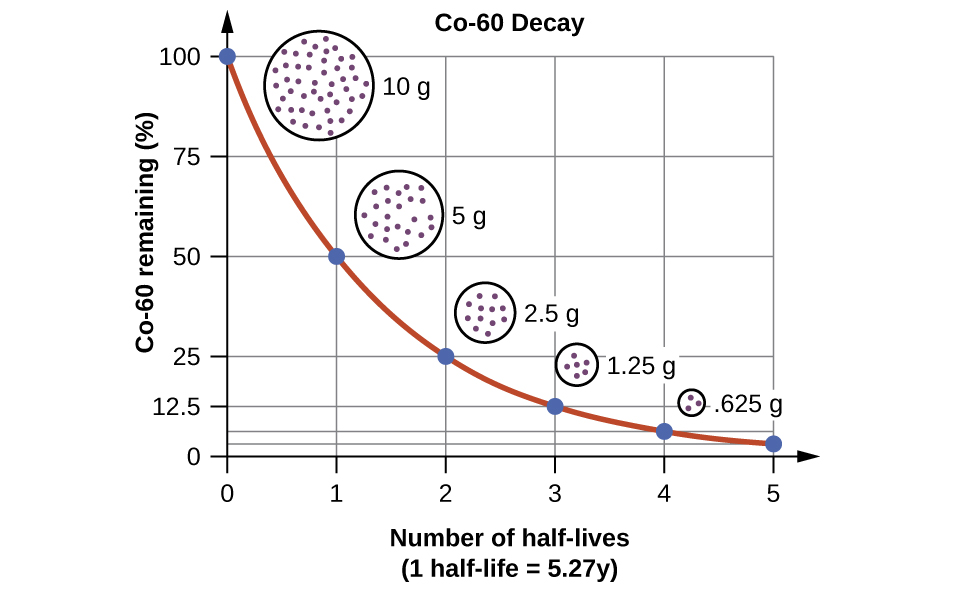
For example, cobalt-60, an isotope that emits gamma rays used to treat cancer, has a half-life of 5.27 years (Figure 12.1). In a given cobalt-60 source, since half of the nuclei decay every 5.27 years, both the amount of material and the intensity of the radiation emitted is cut in half every 5.27 years. (Note that for a given substance, the intensity of radiation that it produces is directly proportional to the rate of decay of the substance and the amount of the substance.) This is as expected for a process following first-order kinetics. Thus, a cobalt-60 source that is used for cancer treatment must be replaced regularly to continue to be effective.
Figure 12.1
For cobalt-60, which has a half-life of 5.27 years, 50% remains after 5.27 years (one half-life), 25% remains after 10.54 years (two half-lives), 12.5% remains after 15.81 years (three half-lives), and so on.
Since nuclear decay follows first-order kinetics, we can adapt the mathematical relationships used for first-order chemical reactions. We generally substitute the number of nuclei, N, for the concentration. If the rate is stated in nuclear decays per second, we refer to it as the activity of the radioactive sample. The rate for radioactive decay is:
decay rate = λN with λ = the decay constant for the particular radioisotope
The decay constant, λ, which is the same as a rate constant discussed in the kinetics chapter. It is possible to express the decay constant in terms of the half-life, t1/2:
The first-order equations relating amount, N, and time are:
where N0 is the initial number of nuclei or moles of the isotope, and Nt is the number of nuclei/moles remaining at time t. Example 12.1 applies these calculations to find the rates of radioactive decay for specific nuclides.
EXAMPLE 12.1.2
Rates of Radioactive Decay
decays with a half-life of 5.27 years to produce(a) What is the decay constant for the radioactive disintegration of cobalt-60?
(b) Calculate the fraction of a sample of the isotope that will remain after 15 years.
(c) How long does it take for a sample of to disintegrate to the extent that only 2.0% of the original amount remains?
Solution
(a) The value of the rate constant is given by:(b) The fraction of that is left after time t is given by Rearranging the first-order relationship Nt = N0e–λt to solve for this ratio yields:
The fraction of that will remain after 15.0 years is 0.138. Or put another way, 13.8% of the originally present will remain after 15 years.
(c) 2.00% of the original amount of is equal to 0.0200 N0. Substituting this into the equation for time for first-order kinetics, we have:
Check Your Learning
Radon-222, has a half-life of 3.823 days. How long will it take a sample of radon-222 with a mass of 0.750 g to decay into other elements, leaving only 0.100 g of radon-222?Answer
11.1 days
Because each nuclide has a specific number of nucleons, a particular balance of repulsion and attraction, and its own degree of stability, the half-lives of radioactive nuclides vary widely. For example: the half-life of is 1.9×1019 years; is 24,000 years; is 3.82 days; and element-111 (Rg for roentgenium) is 1.5×10–3 seconds. The half-lives of a number of radioactive isotopes important to medicine are shown in Table 12.1, and others are listed in Appendix M.
Table 12.2
Half-lives of Radioactive Isotopes Important to Medicine
| Type1 | Decay Mode | Half-Life | Uses |
|---|---|---|---|
| F-18 | β+ decay | 110. minutes | PET scans |
| Co-60 | β decay, γ decay | 5.27 years | cancer treatment |
| Tc-99m | γ decay | 8.01 hours | scans of brain, lung, heart, bone |
| I-131 | β decay | 8.02 days | thyroid scans and treatment |
| Tl-201 | electron capture | 73 hours | heart and arteries scans; cardiac stress tests |
12.1.3 Radiometric Dating
Several radioisotopes have half-lives and other properties that make them useful for purposes of “dating” the origin of objects such as archaeological artifacts, formerly living organisms, or geological formations. This process is radiometric dating and has been responsible for many breakthrough scientific discoveries about the geological history of the earth, the evolution of life, and the history of human civilization. We will explore some of the most common types of radioactive dating and how the particular isotopes work for each type.
12.1.3.1 Radioactive Dating Using Carbon-14
The radioactivity of carbon-14 provides a method for dating objects that were a part of a living organism. This method of radiometric dating, which is also called radiocarbon dating or carbon-14 dating, is accurate for dating carbon-containing substances that are up to about 30,000 years old, and can provide reasonably accurate dates up to a maximum of about 50,000 years old.
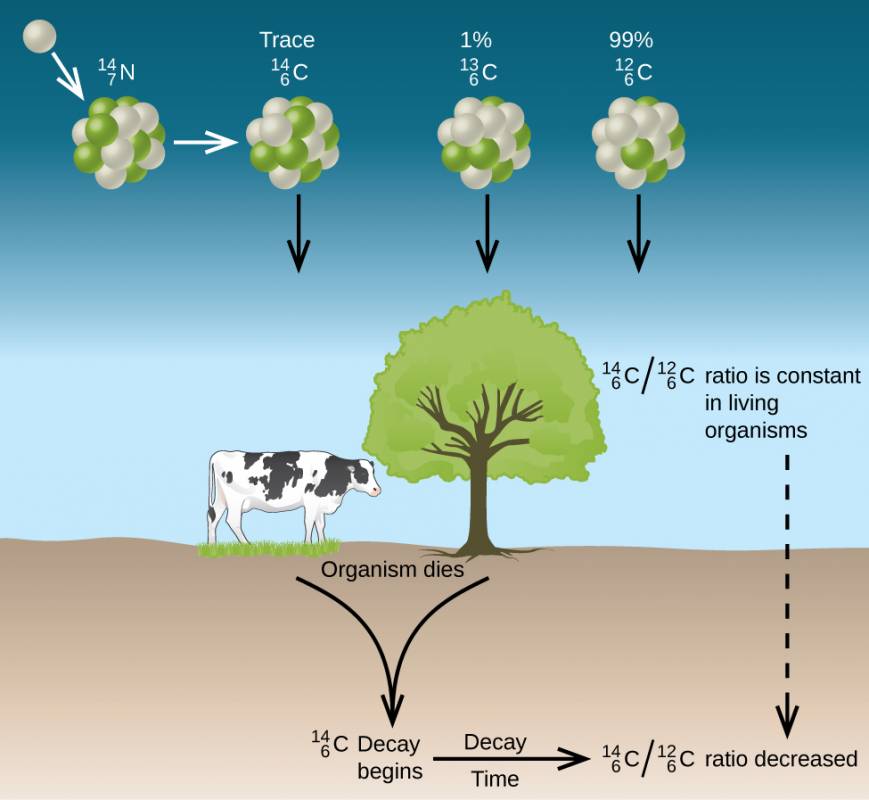
Naturally occurring carbon consists of three isotopes: which constitutes about 99% of the carbon on earth; about 1% of the total; and trace amounts of Carbon-14 forms in the upper atmosphere by the reaction of nitrogen atoms with neutrons from cosmic rays in space:
All isotopes of carbon react with oxygen to produce CO2 molecules. The ratio of to depends on the ratio of to in the atmosphere. The natural abundance of in the atmosphere is approximately 1 part per trillion; until recently, this has generally been constant over time, as seen is gas samples found trapped in ice. The incorporation of and into plants is a regular part of the photosynthesis process, which means that the ratio found in a living plant is the same as the ratio in the atmosphere. But when the plant dies, it no longer traps carbon through photosynthesis. Because is a stable isotope and does not undergo radioactive decay, its concentration in the plant does not change. However, carbon-14 decays by β emission with a half-life of 5730 years:
Thus, the ratio gradually decreases after the plant dies. The decrease in the ratio with time provides a measure of the time that has elapsed since the death of the plant (or other organism that ate the plant). Figure 12.2 visually depicts this process.
Figure 12.3
Along with stable carbon-12, radioactive carbon-14 is taken in by plants and animals, and remains at a constant level within them while they are alive. After death, the C-14 decays and the C-14:C-12 ratio in the remains decreases. Comparing this ratio to the C-14:C-12 ratio in living organisms allows us to determine how long ago the organism lived (and died).
For example, with the half-life of being 5730 years, if the ratio in a wooden object found in an archaeological dig is half what it is in a living tree, this indicates that the wooden object is 5730 years old. Highly accurate determinations of ratios can be obtained from very small samples (as little as a milligram) by the use of a mass spectrometer.
LINK TO LEARNING
Visit this website to perform simulations of radiometric dating.
EXAMPLE 12.1.4
12.1.4.1 Radiocarbon Dating
A tiny piece of paper (produced from formerly living plant matter) taken from the Dead Sea Scrolls has an activity of 10.8 disintegrations per minute per gram of carbon. If the initial C-14 activity was 13.6 disintegrations/min/g of C, estimate the age of the Dead Sea Scrolls.Solution
The rate of decay (number of disintegrations/minute/gram of carbon) is proportional to the amount of radioactive C-14 left in the paper, so we can substitute the rates for the amounts, N, in the relationship:where the subscript 0 represents the time when the plants were cut to make the paper, and the subscript t represents the current time.
The decay constant can be determined from the half-life of C-14, 5730 years:
Substituting and solving, we have:
Therefore, the Dead Sea Scrolls are approximately 1900 years old (Figure 12.3).
Figure 12.4
Carbon-14 dating has shown that these pages from the Dead Sea Scrolls were written or copied on paper made from plants that died between 100 BC and AD 50.

Check Your Learning
More accurate dates of the reigns of ancient Egyptian pharaohs have been determined recently using plants that were preserved in their tombs. Samples of seeds and plant matter from King Tutankhamun’s tomb have a C-14 decay rate of 9.07 disintegrations/min/g of C. How long ago did King Tut’s reign come to an end?Answer
about 3350 years ago, or approximately 1340 BC
There have been some significant, well-documented changes to the ratio. The accuracy of a straightforward application of this technique depends on the ratio in a living plant being the same now as it was in an earlier era, but this is not always valid. Due to the increasing accumulation of CO2 molecules (largely in the atmosphere caused by combustion of fossil fuels (in which essentially all of the has decayed), the ratio of in the atmosphere may be changing. This manmade increase in in the atmosphere causes the ratio to decrease, and this in turn affects the ratio in currently living organisms on the earth. Fortunately, however, we can use other data, such as tree dating via examination of annual growth rings, to calculate correction factors. With these correction factors, accurate dates can be determined. In general, radioactive dating only works for about 10 half-lives; therefore, the limit for carbon-14 dating is about 57,000 years.
12.1.4.2 Radioactive Dating Using Nuclides Other than Carbon-14
Radioactive dating can also use other radioactive nuclides with longer half-lives to date older events. For example, uranium-238 (which decays in a series of steps into lead-206) can be used for establishing the age of rocks (and the approximate age of the oldest rocks on earth). Since U-238 has a half-life of 4.5 billion years, it takes that amount of time for half of the original U-238 to decay into Pb-206. In a sample of rock that does not contain appreciable amounts of Pb-208, the most abundant isotope of lead, we can assume that lead was not present when the rock was formed. Therefore, by measuring and analyzing the ratio of U-238:Pb-206, we can determine the age of the rock. This assumes that all of the lead-206 present came from the decay of uranium-238. If there is additional lead-206 present, which is indicated by the presence of other lead isotopes in the sample, it is necessary to make an adjustment. Potassium-argon dating uses a similar method. K-40 decays by positron emission and electron capture to form Ar-40 with a half-life of 1.25 billion years. If a rock sample is crushed and the amount of Ar-40 gas that escapes is measured, determination of the Ar-40:K-40 ratio yields the age of the rock. Other methods, such as rubidium-strontium dating (Rb-87 decays into Sr-87 with a half-life of 48.8 billion years), operate on the same principle. To estimate the lower limit for the earth’s age, scientists determine the age of various rocks and minerals, making the assumption that the earth is older than the oldest rocks and minerals in its crust. As of 2014, the oldest known rocks on earth are the Jack Hills zircons from Australia, found by uranium-lead dating to be almost 4.4 billion years old.
EXAMPLE 12.1.5
Radioactive Dating of Rocks
An igneous rock contains 9.58 10–5 g of U-238 and 2.51 10–5 g of Pb-206, and much, much smaller amounts of Pb-208. Determine the approximate time at which the rock formed.Solution
The sample of rock contains very little Pb-208, the most common isotope of lead, so we can safely assume that all the Pb-206 in the rock was produced by the radioactive decay of U-238. When the rock formed, it contained all of the U-238 currently in it, plus some U-238 that has since undergone radioactive decay.The amount of U-238 currently in the rock is:
Because when one mole of U-238 decays, it produces one mole of Pb-206, the amount of U-238 that has undergone radioactive decay since the rock was formed is:
The total amount of U-238 originally present in the rock is therefore:
The amount of time that has passed since the formation of the rock is given by:
with N0 representing the original amount of U-238 and Nt representing the present amount of U-238.
U-238 decays into Pb-206 with a half-life of 4.5×109 y, so the decay constant λ is:
Substituting and solving, we have:
Therefore, the rock is approximately 1.7 billion years old.
Check Your Learning
A sample of rock contains 6.14×10–4 g of Rb-87 and 3.51×10–5 g of Sr-87. Calculate the age of the rock. (The half-life of the β decay of Rb-87 is 4.7×1010 y.)Answer
3.7 109 y
12.2 Transmutation and Nuclear Energy
Learning Objectives
By the end of this section, you will be able to:
- Describe the synthesis of transuranium nuclides
After the discovery of radioactivity, the field of nuclear chemistry was created and developed rapidly during the early twentieth century. A slew of new discoveries in the 1930s and 1940s, along with World War II, combined to usher in the Nuclear Age in the mid-twentieth century. Scientists learned how to create new substances, and certain isotopes of certain elements were found to possess the capacity to produce unprecedented amounts of energy, with the potential to cause tremendous damage during war, as well as produce enormous amounts of power for society’s needs during peace.
12.2.1 Synthesis of Nuclides
Nuclear transmutation is the conversion of one nuclide into another. It can occur by the radioactive decay of a nucleus, or the reaction of a nucleus with another particle. The first manmade nucleus was produced in Ernest Rutherford’s laboratory in 1919 by a transmutation reaction, the bombardment of one type of nuclei with other nuclei or with neutrons. Rutherford bombarded nitrogen atoms with high-speed α particles from a natural radioactive isotope of radium and observed protons resulting from the reaction:
The and nuclei that are produced are stable, so no further (nuclear) changes occur.
To reach the kinetic energies necessary to produce transmutation reactions, devices called particle accelerators are used. These devices use magnetic and electric fields to increase the speeds of nuclear particles. In all accelerators, the particles move in a vacuum to avoid collisions with gas molecules. When neutrons are required for transmutation reactions, they are usually obtained from radioactive decay reactions or from various nuclear reactions occurring in nuclear reactors. The Chemistry in Everyday Life feature that follows discusses a famous particle accelerator that made worldwide news.
12.2.2 CHEMISTRY IN EVERYDAY LIFE
CERN Particle Accelerator
Located near Geneva, the CERN (“Conseil Européen pour la Recherche Nucléaire,” or European Council for Nuclear Research) Laboratory is the world’s premier center for the investigations of the fundamental particles that make up matter. It contains the 27-kilometer (17 mile) long, circular Large Hadron Collider (LHC), the largest particle accelerator in the world (Figure 12.4). In the LHC, particles are boosted to high energies and are then made to collide with each other or with stationary targets at nearly the speed of light. Superconducting electromagnets are used to produce a strong magnetic field that guides the particles around the ring. Specialized, purpose-built detectors observe and record the results of these collisions, which are then analyzed by CERN scientists using powerful computers.
Figure 12.5
A small section of the LHC is shown with workers traveling along it. (credit: Christophe Delaere)

In 2012, CERN announced that experiments at the LHC showed the first observations of the Higgs boson, an elementary particle that helps explain the origin of mass in fundamental particles. This long-anticipated discovery made worldwide news and resulted in the awarding of the 2013 Nobel Prize in Physics to François Englert and Peter Higgs, who had predicted the existence of this particle almost 50 years previously.
LINK TO LEARNING
Famous physicist Brian Cox talks about his work on the Large Hadron Collider at CERN, providing an entertaining and engaging tour of this massive project and the physics behind it.
View a short video from CERN, describing the basics of how its particle accelerators work.
Prior to 1940, the heaviest-known element was uranium, whose atomic number is 92. Now, many artificial elements have been synthesized and isolated, including several on such a large scale that they have had a profound effect on society. One of these—element 93, neptunium (Np)—was first made in 1940 by McMillan and Abelson by bombarding uranium-238 with neutrons. The reaction creates unstable uranium-239, with a half-life of 23.5 minutes, which then decays into neptunium-239. Neptunium-239 is also radioactive, with a half-life of 2.36 days, and it decays into plutonium-239. The nuclear reactions are:
Plutonium is now mostly formed in nuclear reactors as a byproduct during the fission of U-235. Additional neutrons are released during this fission process (see the next section), some of which combine with U-238 nuclei to form uranium-239; this undergoes β decay to form neptunium-239, which in turn undergoes β decay to form plutonium-239 as illustrated in the preceding three equations. These processes are summarized in the equation:
Heavier isotopes of plutonium—Pu-240, Pu-241, and Pu-242—are also produced when lighter plutonium nuclei capture neutrons. Some of this highly radioactive plutonium is used to produce military weapons, and the rest presents a serious storage problem because they have half-lives from thousands to hundreds of thousands of years.
Although they have not been prepared in the same quantity as plutonium, many other synthetic nuclei have been produced. Nuclear medicine has developed from the ability to convert atoms of one type into other types of atoms. Radioactive isotopes of several dozen elements are currently used for medical applications. The radiation produced by their decay is used to image or treat various organs or portions of the body, among other uses.
The elements beyond element 92 (uranium) are called transuranium elements. As of this writing, 22 transuranium elements have been produced and officially recognized by IUPAC; several other elements have formation claims that are waiting for approval. Some of these elements are shown in Table 12.2.
Table 12.6
Preparation of Some of the Transuranium Elements
| Name | Symbol | Atomic Number | Reaction |
|---|---|---|---|
| americium | Am | 95 | |
| curium | Cm | 96 | |
| californium | Cf | 98 | |
| einsteinium | Es | 99 | |
| mendelevium | Md | 101 | |
| nobelium | No | 102 | |
| rutherfordium | Rf | 104 | |
| seaborgium | Sg | 106 | |
| meitnerium | Mt | 107 |
Footnotes
1) The “m” in Tc-99m stands for “metastable,” indicating that this is an unstable, high-energy state of Tc-99. Metastable isotopes emit γ radiation to rid themselves of excess energy and become (more) stable.